Different Types Of Quadrilaterals – In geometry, a quadrilateral is a four-sided polygon, which has four edges (sides) and four angles (vertices). The word is derived from the Latin words quadri, the fourth variant, and latus, meaning “side”. It is also called a quadrangle, derived from the Greek “tetra” meaning “four” and “gon” meaning “corner” or “corner”, similar to other polygons (e.g. ptagon). Since “gon” means “corner”, it is called, for example, a quadrilateral or a 4-corner. A quadrilateral with vertices A, B, C, and D is sometimes labeled A B C D.
Quadrilaterals are simple (non-intersecting) or complex (intersecting or intersecting). The fourth simple is soft or soft.
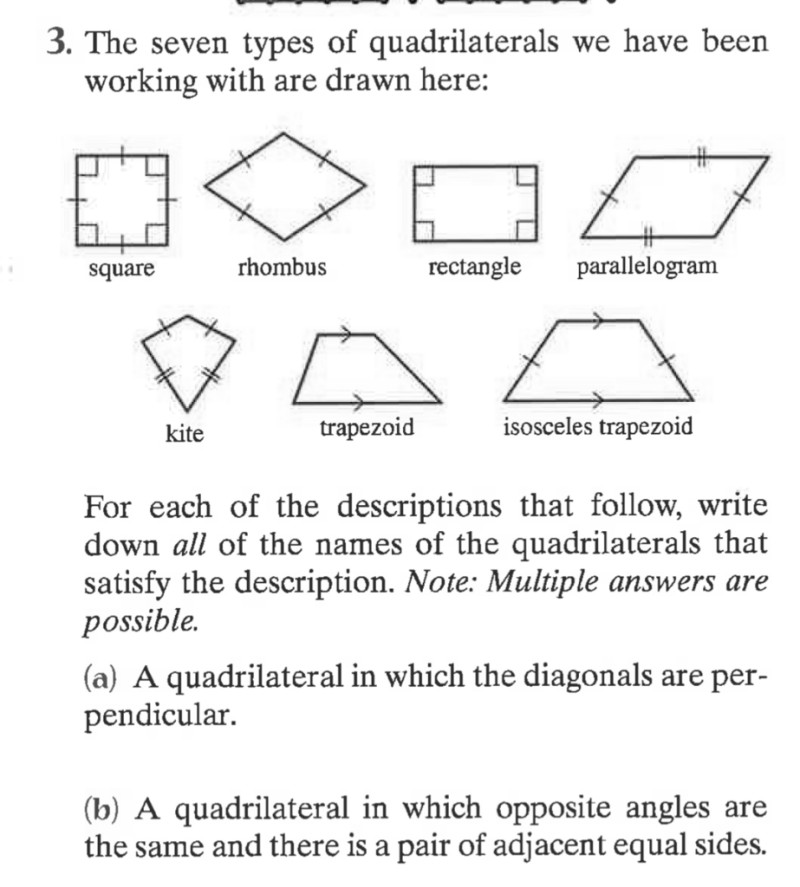
Different Types Of Quadrilaterals

The sum of the internal angles of a simple (and flat) quadrilateral ABCD is 360 arc degrees, i.e.
Trapezium: Definition, Formula, Types & Properties
This is a special case of the formula for the sum of the internal angles of the corner of n: S = (n – 2) × 180 °.
In a convex quadrilateral, all interior angles are less than 180°, and both diagonals are inside the quadrilateral.
In a concave quadrilateral, one internal angle is greater than 180°, and one of the two diagonals is outside the quadrilateral.
A quadrilateral that intersects in various ways is called a quadrilateral, a quadrilateral, a quadrilateral, or a butterfly. In a crossed quadrangle, the four “interior” angles on all sides of the cross (two acute and two reflex, both to the left or all to the right as the picture is drawn) add up to 720°.
Understanding Quadrilaterals:what Is A Polygon 8th Math
Two bimedians of a convex quadrilateral are line segments joining the points of opposite sides.
These meet at the “vertex of the ctroid” of the quadrilateral (see § Strange Points and Lines in Convex Angles below).
The four sides of a convex quadrilateral are perpendicular to the side – through the center of the opposite side.

There are various formulas for the area K of a convex quadrilateral ABCD with sides a = AB, b = BC, c = CD and d = DA.
Intro To Quadrilateral (video)
In the case of orthodiagonal quadrilaterals (eg rhombus, square and kite), this formula reduces to K = p q 2 }} since θ is 90°.
Where the sides are in the sequence a, b, c, d, where s is a semicircle, and A and C are two (actually, any two) opposite angles. This is based on Brahmagupta’s formula for the area of a quadrilateral—wh A + C = 180°.
Another method of area in terms of sides and angles, where angle C is between sides b and c, and A is between sides a and d, is.
In the case of cyclic quadrilateral, the final formula becomes K = a d + b c 2 sin A . }sin.}
Properties Of Quadrilaterals
In a parallelogram, where both pairs of sides and angles are equal, this formula reduces to K = a b ⋅ sin A . .}
Alternatively, we can write the area in terms of the sides and the angle of intersection θ of the diagonals, when θ is not 90 °:
Where x is the distance between the centers of the diagonals, and φ is the angle between the bimedians.

The final formula for a trigonometric surface consisting of sides a, b, c, d and angle α (between a and b) is:
All Kinds Of Measurements For All Kinds Of Quadrilaterals — Krista King Math
Which can also be used for a concave quadrilateral surface (having a concave part opposite the angle α), just by changing the first sign + to -.
The following two methods describe the area in terms of sides a, b, c and d, half of the circle s and diagonals p, q:
K = ( s − a ) ( s − b ) ( s − c ) ( s − d) − 1 4 ( a c + b d + p q ) ( a c + b d − p q ) , }(ac+bd+pq)(ac +bd-pq)}}, }
The first reduces to Brahmagupta’s formula in the case of quadrilateral rotation, since th pq = ac + bd.
Solution: Type Of Quadrilaterals Trapezoid And Parallelogram
The area can also be shown through the two symbols m, n and the diagonal p, q:
K = ( m + n + p ) ( m + n − p ) ( m + n + q ) ( m + n − q ) 2 , }} , }
The area of quadrilateral ABCD can be calculated using vectors. Let the vectors AC and BD form a diagonal from A to C and from B to D. The area of the quadrilateral is th.

Which is half the magnitude of the vector product AC and BD. In two-dimensional Euclidean space, representing the vector AC as a free vector in Cartesian space equal to (x1, y1) and BD as (x2, y2), this can be rewritten as:
What’s A Quadrilateral?!
The following table shows whether the diagonals in some of the most basic vectors bisect each other, whether their diagonals are triangular, and whether their diagonals are of equal length.
Note 1: many trapezoids and isosceles trapezoids do not have vertical diagonals, but there are an infinite number of trapezoids (different) and isosceles trapezoids that have vertical diagonals and not any other quadrilateral.
Note 2: In a kite, one diagonal bisects another. The most general kite has unequal diagonals, but there are an infinite number of (unequal) kites whose diagonals are the same length (and the kite is not any other so-called quadrilateral).
The length of the diagonal in the convex quadrilateral ABCD can be calculated using the law of cosines for any triangle formed by one diagonal and two quadrilateral sides. So
Quiz & Worksheet
P = ( a c + b d ) ( a d + b c ) − 2 a b c d ( cos B + cos D ) a b + c d +cos )}}}}
Q = ( a b + c d ) ( a c + b d ) − 2 a b c d ( cos A + cos C ) a d + b c . +cos )}}}.}
In any convex quadrilateral ABCD, the sum of the squares of the four sides is equal to the sum of the squares of the two diagonals plus four times the square of the line segment joining the centers of the diagonals. So

In 1842, the German mathematician Carl Anton Bretschneider found the following generalization of Ptolemy’s theorem, about the product of the diagonals in a convex quadrilateral.
Quadrilaterals Lesson Module
This relationship can be considered as the cosine law in four dimensions. In the quadrilateral circle, where A + C = 180 °, reduces pq = ac + bd. Since cos (A + C) ≥ −1, this also proves Ptolemy’s inequality.
If X and Y are the bases of the normal rules from B and D to the diagonal AC = p in the quadrilateral ABCD with sides a = AB, b = BC, c = CD, d = DA, th.
In a convex quadrilateral ABCD with sides a = AB, b = BC, c = CD, d = DA and where the diagonals intersect at point E,
E f g h ( a + c + b + d ) ( a + c − b − d ) = ( a g h + c e f + b e h + d f g ) ( a g h + c e f − b e h − d fg )
Triangles And Quadrilaterals
The shape and size of a convex quadrilateral is completely determined by the length of its sides that are parallel to one of the two defined vertices. Two diagonals p, q and four side lengths a, b, c, d of the quadrilateral are connected.
(that is, the four points of intersection of two adjacent angle components are congruent) or congruent. In the latter case, the quadrilateral is a tangential quadrilateral.
In a quadrilateral ABCD, if the two bisectors of angles A and C meet on the diagonal BD, the two bisectors of angles B and D meet on the diagonal AC.

Bimedias of quadrilaterals are lengths joining the centers of opposite sides. The bimedian intersection is the ctroid of the vertices of the quadrilateral.
Solved 10. The Seven Types Of Quadrilaterals We Have Been
The vertices of the sides of the quadrilateral (convex, concave or crossed) are the vertices of the parallelogram called Varignon parallelogram. It has the following features:
Two muscles in a quadrilateral and the line joining the midpoints of the diagonals in the quadrilateral are parallel and both are divided by the point of intersection.
In a convex quadrilateral with sides a, b, c and d, the length of the bisector joining the midpoints of sides a and c is
The length of the bimedian can also be expressed in terms of two different sides and the distance x between the centers of the diagonals. This is possible by using Euler’s quadrilateral theorem in the above formulas. What
Types Of Quadrilateral And Their Properties
Sin A + sin B + sin C + sin D = sin 4 A + B 2 sin A + C 2 sin A + D 2 +sin +sin +sin =4sin } sin }sin }}
Tan Tan
Different types of quadrilaterals and their properties, what are the different types of quadrilaterals, different types of quadrilaterals with their properties, properties of different quadrilaterals, different kinds of quadrilaterals, five types of quadrilaterals, six types of quadrilaterals, special types of quadrilaterals, different names of quadrilaterals, properties of different types of quadrilaterals, 6 types of quadrilaterals, 7 types of quadrilaterals