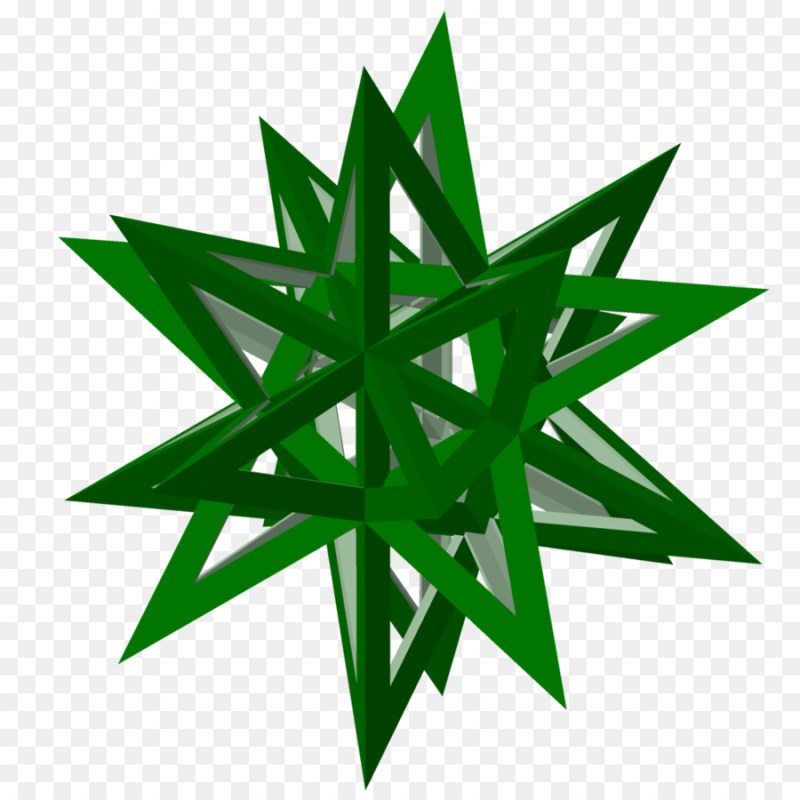
Great Stellated Dodecahedron – IIIF offers researchers a wealth of metadata and media viewing options to compare work in cultural heritage collections. Visit the IIIF page for more information.
This media is in the public domain (no copyright). You may copy, modify, and distribute this work without contacting the Smithsonian. For more information, visit the Smithsonian’s Terms of Service.
Great Stellated Dodecahedron

A regular polyhedron is a polyhedron whose faces are formed by extending the edges of the faces of regular polyhedra. For example, if we extend the sides of a regular pentagon, we can get a five-pointed star or a pentagram. Considering the combination of the twelve pentagrams formed by the twelve pentagonal faces of a regular dodecahedron, we obtain this surface called the dodecahedron in the form of a large star. A regular icosahedron can also be formed by gluing suitable triangular pyramids on the faces – there are a total of sixty triangular faces.
Awesome Star Necklace
The large star-shaped dodecahedron was published by Wenzel Jamnitzer in 1568. It was rediscovered by Johannes Kepler and published in his work Harmonice Mundi in 1619. The French mathematician Louis Poinsot rediscovered it in 1809, and the surface and three associated stars are known as Kepler. – Point solids.
This white plastic pattern with a large star-shaped dodecahedron is marked on one side on a paper label: 43 (/) DIV. A. Harry Wheeler assigned the model number 43 in his diagram and considered it the fourth type of a dodecahedron.
Our collection database is a work in progress. We may update this record based on additional research and reviews. Learn more about our approach to sharing our collection online.
If you want to know how you can use the content on this page, see Smithsonian’s Terms of Use. If you need to request an image for publication or other use, please visit the Rights and Reproductions page.
Uniform Polyhedron Stellation Small Stellated Dodecahedron Truncated Octahedron, Png, 744x1052px, Polyhedron, Area, Cube, Dodecahedron, Great Stellated
Note: Sending feedback is temporarily unavailable when making improvements to the site. We apologize for the interruption. If you have a question about the museum’s collections, please first review our Collections FAQ. If you need a personal answer, please use our Contact page. While a (smaller) dodecahedron is a star shape, it shares the vertex arrangement with the regular dodecahedron, but not the vertex figure or vertex configuration. Apart from the dodecahedron itself, it is the only dodecahedral star with this feature. Its twin, the greater icosahedron, is similarly related to the icosahedron.
If the ptagrammic faces are triangulated, they are topologically related to the triakis icosahedron, the same connectivity of the faces, but the much longer isosceles triangular faces. If instead the triangles are made to invert and carve the central icosahedron, the result is a large dodecahedron.
The large stellate dodecahedron can be formed similarly to the ptagram, its two-dimensional analogue; that is, it is his last star.

This polyhedron can be made as a spherical slab with a density of 7.
File:truncated Great Stellated Dodecahedron With Red Triangle.svg
A large star-shaped dodecahedron (surface geometry); twenty isosceles triangular pyramids arranged like the faces of an icosahedron.
It can be constructed as the third of the three stars of the dodecahedron and is called Wninger’s model [W22].
A cutting operation applied to the large star-shaped dodecahedron produces a series of uniform polyhedra. Cutting the edges into points produces the large icosidodecahedron as a rectified large star-shaped dodecahedron. The process ends with a mating that reduces the original faces to points and produces a large icosahedron.
The great truncated stellate dodecahedron is a degenerate polyhedron with 20 triangular faces from the truncated corners and 12 (hidden) pentagonal faces as truncations of the original faces of the ptagram; the second creates a large inscribed dodecahedron inside and shares the edges of the frame. icosahedron IIIF offers researchers a wealth of metadata and media viewing options to compare work in cultural heritage collections. Visit the IIIF page for more information.
Small Stellated Dodecahedron Jpeg
A regular polyhedron is a polyhedron whose faces are formed by extending the edges of the faces of regular polyhedra. For example, if we extend the sides of a regular pentagon, we can get a five-pointed star or a pentagram. Considering the combination of the twelve pentagrams formed by the twelve pentagonal faces of a regular dodecahedron, we obtain this surface called the dodecahedron in the form of a large star. A regular icosahedron can also be formed by gluing suitable triangular pyramids on the faces – there are a total of sixty triangular faces.
The large star-shaped dodecahedron was published by Wenzel Jamnitzer in 1568. It was rediscovered by Johannes Kepler and published in his work Harmonice Mundi in 1619. The French mathematician Louis Poinsot rediscovered it in 1809, and the surface and three associated stars are known as Kepler. – Point solids.
This cut and folded white paper pattern of dodecahedron in the shape of a large star is marked 6-12-25. It is also marked as: No 43. A. Harry Wheeler assigned the model number 43 in his diagram and considered it the fourth type of a dodecahedron.

Our collection database is a work in progress. We may update this record based on additional research and reviews. Learn more about our approach to sharing our collection online.
Compound Of Small Stellated Dodecahedron And Great Dodecahedron
If you want to know how you can use the content on this page, see Smithsonian’s Terms of Use. If you need to request an image for publication or other use, please visit the Rights and Reproductions page.
Note: Sending feedback is temporarily unavailable when making improvements to the site. We apologize for the interruption. If you have a question about the museum’s collections, please first review our Collections FAQ. If you need a personal answer, please use our Contact page. A convex regular icosahedron is the basis for making this large star-shaped dodecahedron. Star-shaped means “star-shaped”. The icosahedron is transformed into a star-shaped polyhedron by adding twenty isosceles triangular pyramids. A large star-shaped dodecahedron has 12 faces, 20 vertices, and 30 edges.
The convex regular dodecahedron is one of the five regular Platonic solids. The convex regular dodecahedron has three stars, all of which are regular star-shaped dodecahedrons. These are small star-shaped dodecahedron, large dodecahedron. and a large star-shaped dodecahedron. I made the small star shaped dodecahedron in the previous post.https:///2019/11/a-stem-project-small-stellated.html and the big dodecahedron in the previous post.https:///2019/11 /a – stem-project-great-dodecahedron.html Here I will create a large star shaped dodecahedron.
Attention, this pattern requires patience for collage. Installation is not difficult, but drying time is very important. I used Aleene’s Fast Grab Tacky adhesive.
Stellated Dodecahedron Hi Res Stock Photography And Images
Fold the holes of the triangular pyramid. Apply glue to the left side that looks like a triangle.
Glue the triangular pyramid onto the icosahedron. In this photo I show the amount of glue I applied to the icosahedron and a photo of the glue I used.
Glue a string to the middle of the last triangular pyramid. This will allow you to hang the model. Apply glue to the triangular tab and make sure the string extends from the top of the pyramid to the top.

Stellated rhombic dodecahedron, stellated dodecahedron net, great stellated dodecahedron net, stellated dodecahedron sacred geometry, great stellated dodecahedron template, small stellated dodecahedron template, stellated dodecahedron, small stellated dodecahedron, small stellated dodecahedron net, stellated dodecahedron template, stellated dodecahedron origami, great stellated dodecahedron origami